Euler’s totient function counts the number of positive integers up to n that are relatively prime to n, where 1 is considered to be relatively prime to all n. These numbers are called the totatives of n.
The totient function is denoted by \varphi(n).
\varphi(p) = p-1, for a prime p.
Euler’s product formula for the function is :
\displaystyle \varphi(n) = n\prod_{p|n}\Bigl(1-\frac 1p \Bigr),
where p|n are the distinct primes that divide the given number and \prod is the product over them.
For example, 12 = 2^2 \cdot3
\displaystyle \varphi(12) = 12\Bigl(1-\frac12 \Bigr)\Bigl(1-\frac 13 \Bigr)
\displaystyle = 12 \cdot \frac12 \cdot \frac23 = 4
The totatives of 12 are 1, 5, 7 and 11.
\displaystyle \varphi(13) = 13\Bigl(1-\frac{1}{13}\Bigr)
\displaystyle = 13 \cdot \frac{12}{13} = 12
The totatives of 13 are all the numbers less than 13.
\displaystyle \varphi(30) = 30\Bigl(1-\frac12\Bigr)\Bigl(1-\frac13\Bigr)\Bigl(1-\frac15\Bigr)
\displaystyle = 30 \cdot \frac12 \cdot \frac23 \cdot \frac45 = 8
The totatives of 30 are 1, 7, 11, 13, 17, 19, 23 and 29.
The first few values for \varphi(n) :
\begin{array}{c|cccccccccccc} n&1&2&3&4&5&6&7&8&9&10&11&12 \\ \varphi(n)&1&1&2&2&4&2&6&4&6&4&10&4 \end{array}
A graph of the first thousand values :
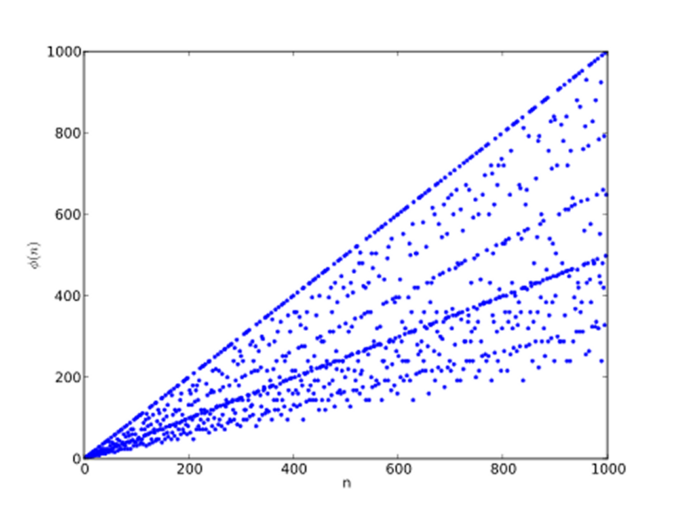
Pietro Battiston (it:User:Toobaz) [CC BY-SA]
\varphi(mn) = \varphi(m)\varphi(n)
This aids speed of computation, for example,
\varphi(35) = \varphi(5)\varphi(7) = 4\cdot6 = 24
Another property of the function is the prime power argument :
\varphi(p^\alpha) = p^\alpha-p^{\alpha-1},
for prime p and \alpha \geq 1.
For example,
\varphi(2^6) = 2^6-2^5 = 64-32 = 32
and
\varphi(5^3) = 5^3-5^2 = 125-25 = 100.
Gauss established another identity that relates the divisors of n to n via the formula
\displaystyle \sum_{d|n} \varphi(d) = n
where d|n are the divisors of n and \sum \varphi(d) is the sum of the totients of the divisors.
For example, the divisors of 12 are 1, 2, 3, 4, 6 and 12 which have totients 1, 1, 2, 2, 2, 4.
Now, 1 + 1 + 2 + 2 + 2 + 4 = 12 so
\displaystyle \sum_{d|12} \varphi(d) = 12
For a prime number the sum is very simple. For example, the divisors of 13 are 1 and 13 which have totients 1 and 12 and 1 + 12 = 13.
Euler generalised Fermat’s Little Theorem.
Euler’s Theorem states :
a^{\varphi(n)} \equiv 1\pmod n
where a and n are relatively prime (gcd(a, n) = 1).
The special case where n is prime is Fermat’s Little Theorem :
a^{p-1} \equiv 1 \pmod p
© OldTrout 2018