Introduction
The “flat earth” people like to disseminate their woeful ideas. Therefore, let us spend some little time to think about the earth that we all live upon. In particular its spherical (well, almost) shape and its rotation. Even with basic mathematics we can see that the earth is a rotating sphere. Tilted to one side but that’s how I am most days!
So, let’s start with a flat earth.
Foucault’s Pendulum
In 1851 a French chap named Léon Foucault had a very clever idea – to use a pendulum to prove the earth’s rotation. That’s quite remarkable. As an amateur astronomer I can observe a star moving in the night sky. But what is moving? The stars or us? Well, everything is. But the apparent motion of Foucault’s pendulum can only be explained by the earth’s rotation. Here’s why:
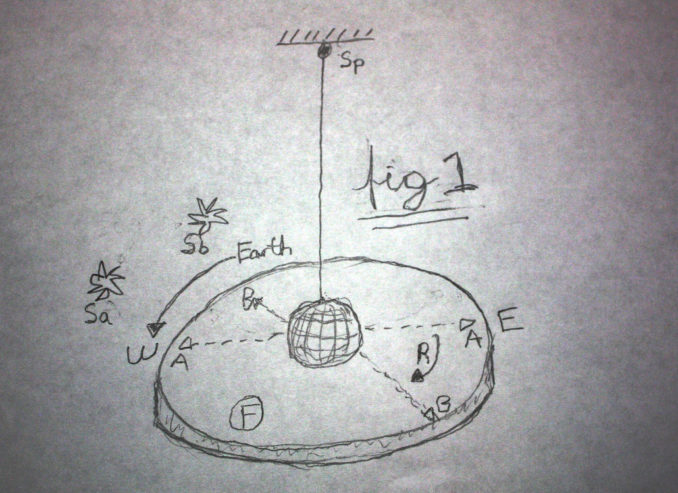
We have a flat earth (F) rotating in the direction indicated – counter clockwise as viewed from the north pole, i.e rotating from west (W) to east (E). The pendulum’s support (Sp) is also “attached” to our flat earth.
Let’s start swinging that pendulum between the two points marked (A). If our flat earth is not rotating our pendulum will continue to swing between those two points until friction says otherwise.
But that’s not what happens. After (let’s say) an hour an apparent clockwise drift of the pendulum occurs in the direction (R). It looks like the pendulum has rotated clockwise and it is now swinging between the points marked (B). Which to an observer on our flat earth it has.
If we observe a distant star (Sa) and set our pendulum pointing toward it, that star will appear to have moved in a clockwise direction (Sb). Of course, it’s the same star but we (on our flat earth) have changed position relative to it. The very heavy mass of the pendulum refuses to change direction, thus it keeps pointing to the star. This resistance is called inertia. As the earth rotates the ball isn’t…err…playing ball!
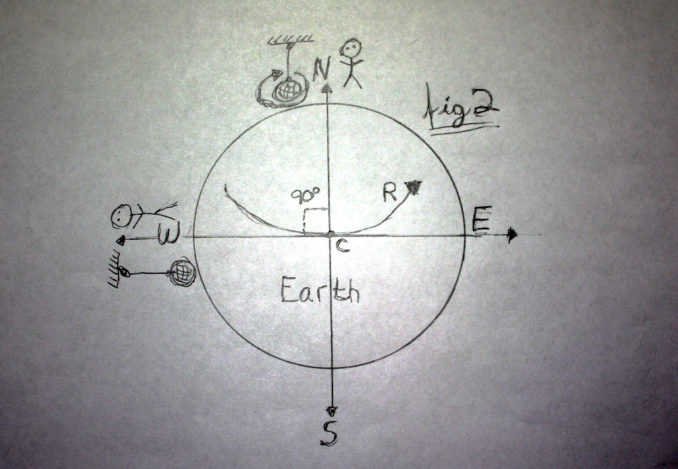
This experiment can also prove that earth is a sphere (otherwise all of mathematics breaks down!). The earth is rotating in the direction (R) – from west to east. If I stand at the north pole the pendulum would appear to rotate (or drift) clockwise, as indicated. At the south pole it would be anticlockwise. If I’m at the equator there would be no drift. And some simple mathematics will explain why.
The time it takes our earth to complete one full west to east rotation is 23 hours and 56 minutes. We’ve removed the addition of solar orbital time, i.e what clocks, watches and sun dials measure to show midday. Foucault’s pendulum cares not for it.
The simple formula is: 23.93 hours divided by the sine(of the latitude). This is how long it takes the pendulum to complete a 360 degree rotation. So at the north pole it’ll take 23.93 hours and the same goes for the south pole. At the equator nothing happens – as we get a divide by zero error! The latitude at the poles is 90 degrees and at the equator zero.
I’m at about 53 degrees north. So the pendulum would take about 30 hours. Foucault was at 48 degrees so about 32 hours.
A Simple Demonstration (with plumbing solder!)
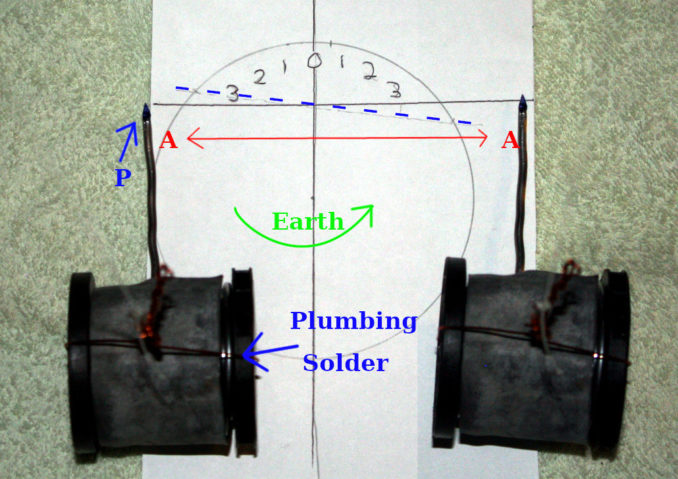
I did not think that this experiment would work as it’s on such a small scale. In the above photo (a double exposure – it’s the same pendulum) I’ve suspended a roll of plumbing solder from my lab ceiling with old nylon guitar strings. It’s swinging between the points marked (A). The length of the suspension string is about eight feet and the oscillation distance is about five inches.
Now, if the earth is not rotating (as indicated), over time nothing will happen. But…
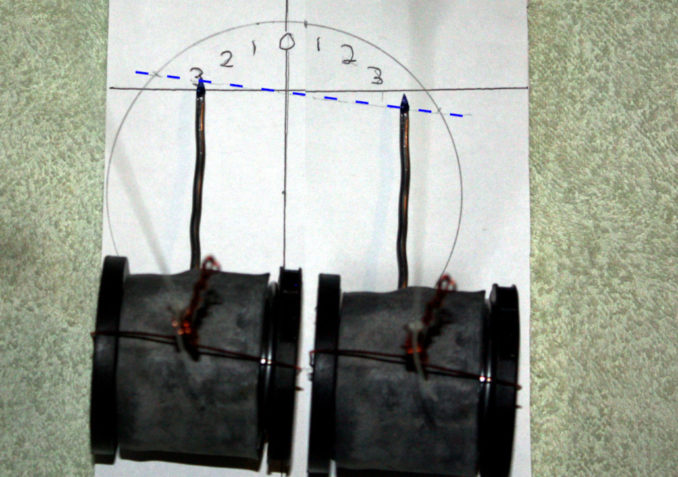
After about twenty minutes you can clearly see that the pointer (P) appears to have changed its position. Of course, it has not. We have moved to the east while it still swings in the same direction.
The dashed blue line shows what I calculated the pendulum’s path to be after 30 minutes.
I’ve conducted this experiment well over a dozen times. I observed the same results every time. Until 3 AM at one point. Always the same motion over the same time period.
Postscript
I know some of you are wondering, “where is the gyroscope?”. I’m splitting the article into two parts. First, because of the length. Second, personal reasons.
If I don’t complete the second part (b) by the new year I wish all puffins a very merry Christmas.
© text & images Doc Mike Finnley 2021



