photo: featured_image.jpg
Introduction
Note: This article uses Mathjax script. Some script blocking web-browser plugins can prevent the mathematics from been rendered correctly. DM
In the first part we looked at how to make a simple slide rule to perform addition and subtraction. But, if we want to reach the moon we’re going to need something more than just addition and subtraction. Let’s look at the basic building blocks of a battery free scientific calculator.
Multiplication, Division and Logarithms
John Napier, a Scottish mathematician, is the first to be credited with the discovery of logarithms. I’m sure that quite a few Puffins remember their dreaded mathematics lessons. Memorising multiplication tables and learning long division. You’re not alone.
Something magic happens when we turn our “linear scales” into “non linear scales” or make them logarithmic. Let’s explore how the genius of Mr Napier accomplished this task.
First some maths (Yay!, says no one). You can skip this section if you wish and (trust me) you’ll still be able to work a slide rule. I thought I’d include it for completeness.
Indices And Powers
Our addition/subtraction slide rule started with the number 0. A logarithmic slide rule starts with the number 1. Why?
Any number raised to the power of 0 = 1. If I raise, say, the number 2 to the power of 3 we get: 2X2X2=8.
Now think. What happens if we raise the number 2 to the power of 0?
Look at this simple equation:
{x^n \over x^n} = 1
It simply states that any number divided by itself is equal to 1, so long as x is not equal to zero. And quite rightly so, otherwise we get infinity.
{x^a \over x^b} = x^{a-b}
The above equation is called the “rule of indices”. If a and b are of equal values, say 3, then the equation has to equal 1 so long as x is not 0:
{x^3 \over x^3} = x^{3-3} = x^0 = 1
Calculating A Logarithmic Scale

We can all draw a ten centimetre line (or inch, it doesn’t matter so long as the spacing is equal) and mark each point from one to ten. In fact, that is all we need to calculate. Once we know the ratios between 1 and 10 we can convert our linear number scale into a logarithmic one.
First we need to decide on the resolution of our scale. We have to pick a number that is very close to the number 1. We can’t have the number 1 because 1 X 1 X 1 X 1… = 1. So I’ve chosen the number 1.1 which is pretty coarse but it will demonstrate how this works. Ideally you’d use 1.01 or better still 1.001, but I’m not writing out a table of nearly 2400 numbers!
You can easily see from the above table how our powers are calculated. We start with 1.1^0 = 1. We then move on to 1.1^1 = 1.1X1 = 1.1 and so forth until we get to 1.1^24 = 9.9 which is very close to 10.
So we’ve calculated 25 powers and all we need to do is “squash” those powers into our number line from 1 to 10. Easy.
First let’s assume our number line is measured in centimetres. If we look at our table you can see that 1.1^7 = 1.95 which is very close to the number 2.
Now we simply work out how many centimetres along our scale we need to mark the number 2:
{10 \over 24} \times 7 = 2.9 cm
Which is nearly 3 cm. Doing the same for the number 3 we find a power of 12 and, using the above formula, that equals 5 cm and so on until we get to 10. Want to know where 2.5 is? Even easier! You simply revert back to a liner scale. So 2 = 3 cm and 3 = 5 cm therefore 2.5 is simply half way between 2 and 3 or 4 cm.
This is what our new scales look like:

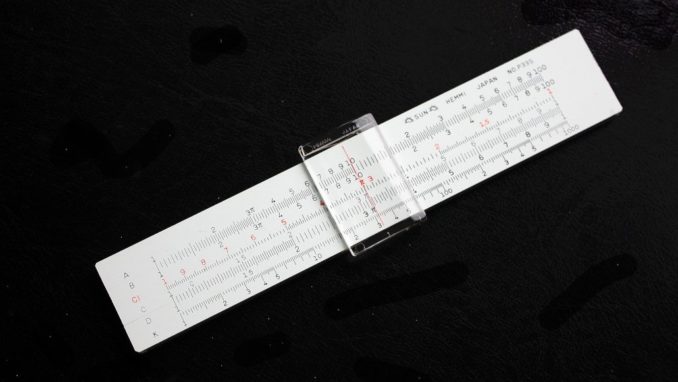
Now we know how to scale 1 to 10 we can easily scale 10 to 100 or 100 to 1000. If you look at the top photo of this article I’ve positioned the cursor over the number 10. Notice how it’s exactly half way along the rule. The distance between 1 and 2 is exactly the same as 10 and 20. Simple but brilliant!
I’ve added a third scale which is linear and marked in tenths. This is what I call the “ironic scale” of a slide rule. Even though it’s linear it’s actually computing the logarithm to base 10 of the A and B scales. For example: log10(5) = 0.7 This is very useful when calculating roots and powers of numbers other than 2 and 3.
In the above photo I’ve set the B rule’s 1 underneath the A rule’s 2. We now have multiplication and division by 2. Reading from left to right: A->B->A we get (for example) 2(A) X 3(B) = 6(A). Inversely, if we read right to left we get (again, for example) 8(A) / 4(B) = 2(A).
This leads us onto calculating fractions really easily. For example: calculate the reciprocal of 2 or 1 / 2. Read B divided by A.
Let’s find the decimal value of 3.5 / 7 = ? You have to learn how to shift your decimal point and remember it at each stage of what you’ve calculated. It’s the art of the slide rule. In this case we want to know the decimal of 1/2. Simply look at the the number 10 on the A scale (10 is your friend) now look at the B scale and we get 5.
Because we’ve shifted the decimal point one place to the right (10.0 instead of 1.0) we need to shift it back. So the answer is not 5.0 but 0.5.The art of the slide rule! Therefore, 3.5 / 7 = 0.5. Remember: It’s a parallel computer.
Conclusion
This has been one of my most difficult two part articles to write with nearly twelve months of thought. I hope that some of you have learned a little about this brilliant device.
I might write a few follow up articles where I’d introduce such concepts as using logarithms to calculate powers and roots, using trigonometry to calculate the height of a building or even measuring how far you’d have to drill, in a straight line, to get to the Earth’s axis given your latitude – just using a slide rule.
The next time you drive your car or get onto a train/plane or, indeed, use your computer, remember the slide rule and that such a simple and humble device has brought us this far.
© text & images Doc Mike Finnley 2023



