Or : Multiplying by i.
The beginning of complex numbers is often attributed to Cardano (1501 – 1576). Complex numbers provide a solution to the equation x^2 = -1.
They are an extension of the number system from \Bbb R (the real numbers) to \Bbb C (the complex numbers).
Complex numbers take the form :
z = a + bi,
where a,b are real numbers and i = \sqrt -1.
The normal rules of arithmetic and algebra apply with the additional rule that :
i^2 = -1
When two complex numbers are multiplied together the real numbers, a and b, act as scalars and the imaginary number, i, acts as a rotation about the origin, 0, (counter-clockwise from the positive real axis). Multiplication by i on its own leads to a rotation of \pi/2 radians in the complex plane.
\begin{align}1\cdot i & = i & = i\\ i\cdot i & = i^2 & = -1\\ -1\cdot i & = i^3 & = -i\\ -i\cdot i & = -i^2 & = 1 \end{align}
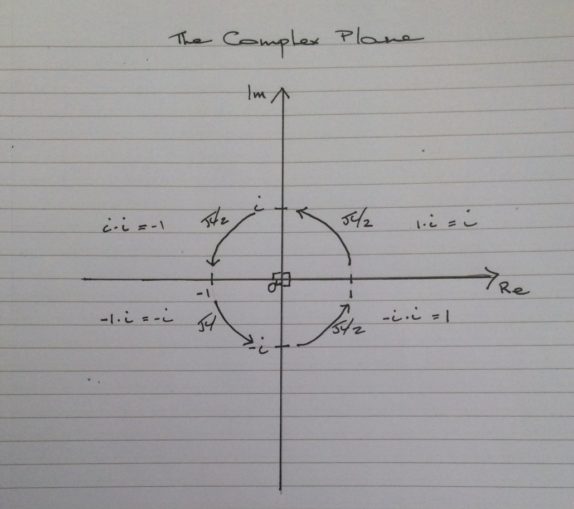
Starting at any point on the real axis, (Re), and repeatedly mutilplying by i traces larger or smaller circles.
The multiplication of two complex numbers gives another complex number.
Let :
z = a + bi and w = c + di
Then :
\begin{align} zw & = (a + bi)(c + di)\\ & = ac + bdi^2 + adi + bci\\ & = ac-bd + (ad + bc)i \end{align}
Taking our starting point as one again, this time we multiply by 1 + i repeatedly.
\begin{align}1(1 + i) & = 1 + i\\ (1 + i)(1 + i) & = 2i\\ 2i(1 + i) & = -2 + 2i\\ (-2 + 2i)(1 + i) & = -4\\ -4(1 + i) & = -4-4i\\ (-4 – 4i)(1 + i) & = -8i\\ (-8i)(1 + i) & = 8-8i\\ (8 – 8i)(1 + i) & = 16 \end{align}
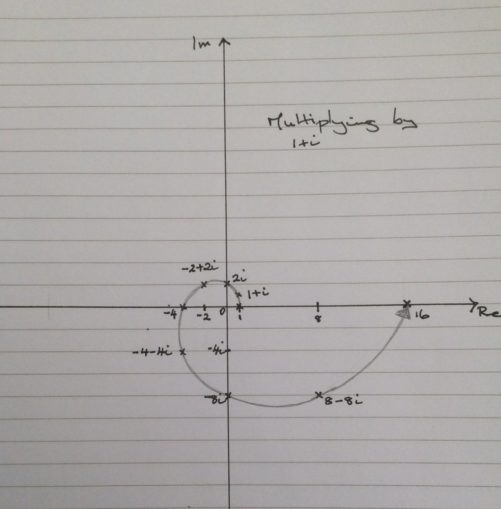
We still have a rotational locus but now in the form of a spiral.
Each multiplication by 1 + i leads to a rotation by \pi/4 and an increase in distance from the origin by \sqrt 2.
This is because the point 1 + i is at a distance \sqrt 2 from the origin, 0, (by Pythagorus) and the angle that this point makes to the positive real axis is arctan (1/1) = \pi/4, where arctan is the inverse of the tangent function.
We look at an example.
Let :
z = 3 + 4i and w = 5 + 2i
Then :
zw = 7 + 26i,
by the above formula.
We can break it down to see what is happening.
(3 + 4i)5 = 15 + 20i
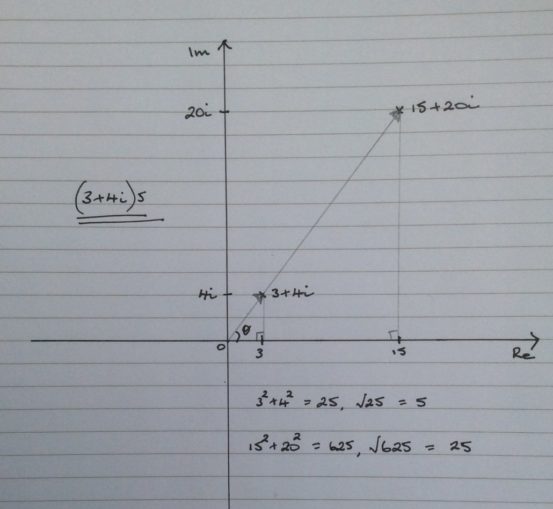
The point has been scaled up by a factor of 5 and the angle is left unchanged.
(3 + 4i)2i = -8 + 6i
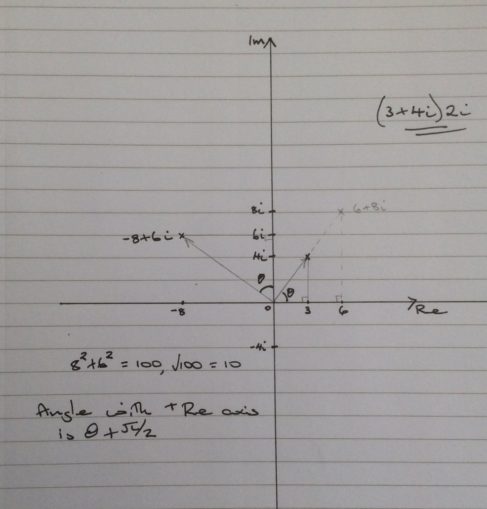
The point has been scaled up by a factor of 2 and rotated by an angle of \pi/2.
The addition of these two parts gives us the point 7 + 26i
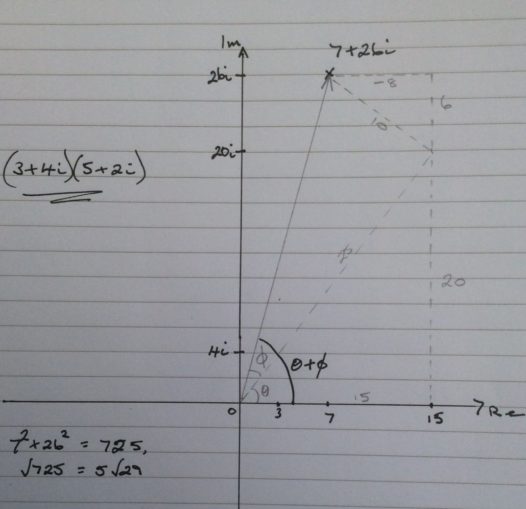
In terms of distances and angles (the polar representation) :
z = (r_1, \theta), w = (r_2, \phi) and zw = (r_1r_2, \theta + \phi).
z = 3 + 4i = (5, \theta), where \theta = arctan(4/3) \approx 0.9273 radians \approx 53.13^\circ
w = 5 + 2i = (\sqrt29, \phi), where \phi = arctan(2/5) \approx 0.3805 radians \approx 21.80^\circ
zw = 7 +26i = (5\sqrt29, \theta + \phi), where\theta + \phi =arctan(26/7) \approx 1.3078 radians \approx 74.93^\circ
Next time we will rotate in three different directions using the quaternions.
© OldTrout 2019



